Wanted: Dead or Alive

Apéry’s constant is simply the value of the Riemann Zeta Function at 3 .

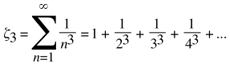= 1.2020569...

This constant is named for Rodger Apéry who proved it was irrational in 1977.

The Riemann Zeta Function

The Riemann Zeta Function in general is:

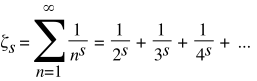
Developed by Bernhard Riemann, this function helps predict the distribution of prime numbers through something called The Riemann Hypothesis. So, maybe the prime numbers are not as unpredictable as we previously thought! Although the hypothesis has not yet been proven, it works so well that mathematicians are quite certain that it’s true.

Intriguing Tidbit

Apéry’s constant shows up unexpectedly in a variety of mathematical places, such as in the solution to various complicated finite integrals, sums, and combinations of the two. We're not going to list them here as they are quite numerous and mildly frightening to the amateur hobby mathematician.

On the otherhand, Apery's Constant has an interesting application to Number Theory. 1/ζ(3) is the probability that three randomly chosen whole numbers are relatively prime. This means that about 83% of the time, three random whole numbers have no factors in common greater than 1. It's really amazing that we are able to supply this probability, given the infinite nature of the numberline.