Double Trouble

With the log_e2 at our side, we can find how long it takes for something to double in size. This could be anything from cell growth or population growth and decay, to the growth of money and the effects of inflation. We will explore what this number is and how it works.

WHAT IS IT?

The natural logarithm (sometimes ln for short) is just a logarithm with base e, the exponential constant. log_e2 in particular is equal to 0.69314718… It’s an irrational and transcendental number with some interesting identities:

• 
• 
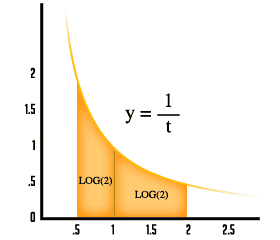
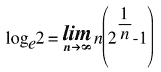
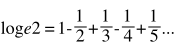
  What happens when you rearrange the terms of this sum? Find out in Playing With Infinity
• The area under the curve 1/x from ½ to 1 is log_e2, and so is the area from 1 to 2.
• Choose a random integer n and look at all its prime factors. The probability that the largest one is greater than 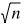is log_e2.

Why Do We Love It?

It is often useful to find a quick rule to estimate things, like how long it would take for your money or population to double at a certain rate. log_e2 can help us out with this! But first, we have to look at how money and population grow in general.

Modelling Growth

Growing population or money can be simply and accurately modeled by a constant growth rate that is compounded every period. OK, so maybe that sounds a bit complicated and scary, but it's really not! Let's work through an example.

A Growing Example

Say you have a annual growth rate of 5% per year. This means that at the end of the first year you get back the original amount plus an extra 5 percent. At the end of the next year you get another 5% on not only the amount you started with, but also the interest you received in the previous year. We call this compound growth, because you earn interest upon interest upon interest! Since compound growth is sometimes more difficult to work with, we can use exponential growth instead as an easy approximation.

Double or Nothin'

OK so now that we've covered the basic model, we can figure out how long it takes for money or population to double. First, consider the equation for exponential growth:

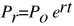
Po is initial pop, Pr is final pop, e is 2.7182..., r is rate, and t is time.

By setting the initial population to 1 and the final population to 2 (effectively doubling our population or money):

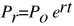
and rearranging:

log_e2 = rt

t = log_e2 / r

we get that t equals log_e2/r. So, the time it takes for your initial amount to double is approximately log_e2 divided by the rate.

The Rule of 70

This is where the rule of 70 (sometimes the rule of 72) comes from. Bankers and financial advisors use this rule to find how long it takes your money to double.

Since log_e2 is approximately .693, they just round it up to .7, which is 70%. So, if you take 70 and divide it by the rate percentage, you get the doubling time of your money.

For instance, if your rate is 5%, 70/5 = 14. So at 5% annual interest rate, it will take about 14 years for your money to double. The exact answer is actually 14.2067, but our rule gets us a very close answer very quickly!

Sometimes 72 is used instead of 70, because it gives a better approximation for higher rates and has more whole numbers that divide evenly into it.