1.41421356237309504880168872420969807856
Be There or be Square!
The square root of 2 is the positive real number that satisfies x2 -2=0. It is most simply the number whose square is 2.
It is also the diagonal length of a right triangle who's side lengths are both 1. We can prove this without even using Pythagoras' theorem. Check out the animation below. The area in the middle is C2.
Notice that when the triangles move, we can see that the middle area must also be the sum of two squares both of area 1. Thus, C2=2. This means that C must be root 2!
Interesting equalities:
- √2= 2sin(π/4)
- √2= e.5ln(2)
Root 2 is Discovered!
The idea of the square root of 2 first appeared sometime before 600 BCE in an ancient Indian math text called the Sulbasutras, written by the Vedic Hindu scholars. Root 2 was the first surd (root) ever discovered. They called root 2 “dvi-karani”, the Sanskrit word which literally means “that which produces 2”. The scholars came up with a pretty good estimation of root 2, correct to 5 decimal places:
1 + 1/3 + 1/(3*4) - 1/(3*4*34) = 577/408 ≈ 1.414215686
Later, around 430 BCE, tradition has it that Pythagoras determined that a square, whose sides are length 1, must have a diagonal of length root 2. As a result, root 2 is sometimes referred to as Pythagoras’ constant.
Mad Influence!
Root 2 has expanded our number system past simply adding, subtracting, multiplying, and dividing whole numbers. Before its irrationality was discovered, the Greeks believed that every number could be expressed as a combination of whole numbers with those four operations. Root 2 broke the mold and showed us that there were more numbers than we ever imagined existing.



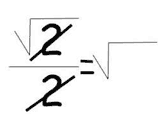

![[Show Animation]](../../images/main/show.gif)