The Musical Series

When we’re talking about music, the harmonic series refers to the series of ratios of frequencies of the harmonics on any given note. This series of ratios (1:1, 1:2, 1:3, 1:4, 1:5…) is important for how music developed, and gives the amazing mathematical series its name.

Harmonics

Whenever a note is played, you don’t just hear one note. You hear the main note, called the fundamental, but also a series of harmonics (also called overtones). Harmonics are notes that are present, but sound higher and fainter than than the note you are actually playing. This is why low sounds are described as ‘rich’, while high notes sound ‘thin’; we can hear fewer harmonics over the high notes, because they quickly get too high for us to hear.

enter the harmonic series

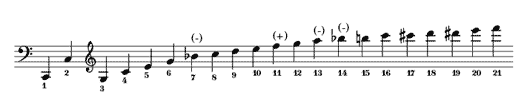
The image shows the harmonics that you hear when a low C is played. The first note is the fundamental, the second harmonic has twice the frequency of the fundamental, the third has three times and so on. The notes with a (+) or (-) over them are out of tune with the note usually played on that note on the staff. You can see how the interval between the notes gets smaller the higher the overtones.

The ratio of the frequencies of these harmonics to the fundamental are in ratios of 1:1, 1:2, 1:3, 1:4… the fractions from the harmonic series! The second harmonic has twice the frequency of the fundamental; the third has three times the fundamental and so on. We perceive pitch logarithmically (for more on this see Light and Sound), and so the intervals get smaller between the harmonics the higher we get. The interval between the fundamental and the second harmonic is an octave (eight notes in a scale), between the second and third interval is a 5th, between the next two is a fourth and so on, until the intervals are too close together to notice a difference.

The Growing Musical Scale

Harmonics allow us to have more than one note in music. At the start of human history, music was just chanting, with only one note. Eventually people became aware (at least subconsciously) of the harmonics of the notes they were singing, and added them to their music. So cavemen might have sung only in octaves, or with a ‘scale’ of three or four notes. The pentatonic scale, with five notes, is present in folk music from around the world. Modern music uses scales with seven main notes, based on one invented by the Greek mathematician Pythagoras.

It’s not all harmony

In reality the intervals we hear in most music today aren’t directly based on harmonics, and if you compare their frequencies you won’t get the nice ratios of the Harmonic Series. This is because we gave up the ‘rationality’ of the old scales to make a system of tones that had exactly the same ratio in frequency to each other. This system is called the 'twelve-tone scale' or 'equal temperament' and was invented by taking an octave (the most basic interval, based on the second harmonic) and dividing it into twelve equal spaced (logarithmically) notes. The ratio between each semitone (the smallest interval) is 1:¹²√2. To get a 5^th we move up 7 semitones. This gives us a ratio of 2:2.99663636... instead of 2:3, but that's not a difference most people can hear. This isn't a very nice ratio, but it makes all the notes even, which makes transposing between keys a lot easier.

Justice for intonation

The notes in this scale approximate the simple ratios of the old scales, and make tuning instruments like pianos simpler, but it does sacrifice some of the resonance that comes from the simple ratios of the harmonic series. The difference isn't obvious to most people, but musicians like to adjust their tuning when they can. Instruments that have a continuous range can adjust their tuning to the key they are playing in, and even some piano players make adjustments. Any tuning systems based on simple ratios is called just intonation. In recent years, some musicians have even returned to early scales, or invented their own along with new instruments.