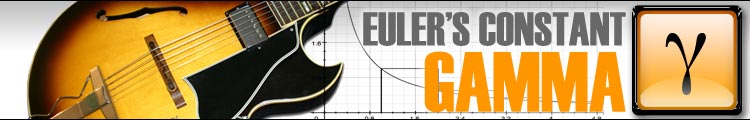
0.5772156649015329
The Difference Between Harmony and Nature
Euler's constant gamma is named for Leonhard Euler, the mathematician who discovered it in 1735. It is represented by the greek letter γ, and is defined as:
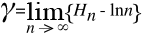
The first step to understanding gamma is to understand its parts. One part of the limit is ln n (also written logen). It is an easily graphible function, and has its own page entitled The Natural Logarithm, accessible from the main page. The other term in the limit is the harmonic series Hn.
The Harmonic Series
The following sum is the harmonic series.
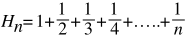
It is called harmonic because of its intimite relation to the harmonics found in music.

The Sound of Harmony
Consider the harmonics of a vibrating string. The first or fundamental harmonic is the whole string vibrating back and forth. The second harmonic vibrates in halves, and the third harmonic vibrates in thirds.
So the wavelengths of the harmonics of a string are proportional to 1, 1/2, 1/3, 1/4, and so forth, which are the numbers in the harmonic series.
To see what a string looks like when the first five harmonics vibrate at once, go to 'Standing waves'.
Flying Off the Handle
The first thing to note about this series is that even though we are adding smaller and smaller fractions together, as n gets larger, so does the sum. In fact, if we let n go to infinity, Hn also goes to infinity. In math speak, we say that the limit of Hn as n goes to infinity doesn’t exist, because Hn never approaches a specific number.
Checking it Out
If you start figuring out the sum yourself, maybe this divergence of Hn off to infinity isn’t so obvious. Although Hn goes to infinity as n goes to infinity, it does this very very slowly.
If we sum the first four terms we get about 2.083, but it takes a total of 100 terms to get to around 5.187. After 1000 terms we reach a mere 7.486, and after a million terms we are only at about 14.392. It would take about 1.5*1043 terms to get a sum larger than 100. That’s huge! So, how do we know that this sum never settles down? See a divergence proof.
Playing Dodgeball
Another interesting property of Hn is that aside from H1, Hn dodges all the integers on its run towards infinity. In fact, aside from H1, H2 and H6, Hn is always a non-terminating decimal.
Back to Gamma
So H∞ is an infinite quantity and so is ln ∞. What’s amazing is that when we subtract them, we end up with a constant. This constant is gamma! So, we can think of gamma as the difference between two infinite things.
Gamma can also be viewed as the error term between Hn and ln n, because the natural logarithm is a very good approximation of the harmonic series. Looking at the graph below, the area of the entire most leftward box is H1. The area of the second box plus the first box is H2, and so on. So the sum of the areas of the first n boxes is Hn. These boxes continue indefinitely to the right. Also appearing below is the quantity ln n. The golden area under the smooth 1/x curve in the first box is ln 2. The golden areas of the second box plus the first box is ln 3 and so on to infinity.
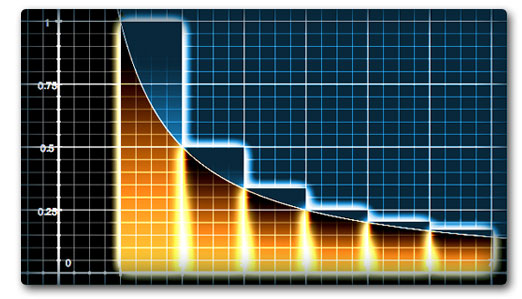
So, as n goes off to infinity, the area of the boxes and the golden area under the curve get really close together. They are so close in fact, that we end up having only a finite error area of blue pieces sticking up above the curve, even though we go to infinity. The areas of these blue pieces are the error terms, and all of these error terms add up to gamma.